Laws of radioactivity
The behavior of radioactive materials and radiation emissions are governed with the fundamental rules of radioactivity. The spontaneous emission of radiation from specific unstable atomic nuclei is referred to as radioactivity.
Rutherford and Soddy studied the phenomenon of radioactivity in detail and formulated the following laws, known as the laws of radioactivity or the laws of radioactive decay.
First of the Laws of Radioactivity:
Radioactivity is a spontaneous phenomenon, and one cannot predict when the nucleus of a particular atom in a given radioactive sample will undergo disintegration.
Second of the Laws of Radioactivity:
When a radioactive atom disintegrates, either an alpha particle (the nucleus of helium) or a beta particle, (an electron) is emitted. The new atom, so formed, is called The nucleus may emit a gamma-ray photon. In cases where the nucleus is left in an excited state or emits the alpha or beta particle further, both alpha and beta particles are never emitted simultaneously. It may also be pointed out that a radioactive atom can never emit more than one alpha or beta particle at a time.
Third of the Laws of Radioactivity:
The emission of an alpha particle by a radioactive atom results in a daughter Atom whose atomic number is two units less and whose mass number is four units less than that of the parent atom.
Due to an alpha-decay, the transformation of the radioactive parent’s atomic nucleus ZXA into the daughter atom (Z-2YA-4) minus 4 may be represented as below.
ZXA ——-> 2He4 + Z-2YA-4
Fourth of the Laws of Radioactivity:
The emission of a beta particle by a radioactive atom results in a daughter atom, whose atomic number is one unit higher but whose mass number is the same as that of the parent atom.
Due to beta-decay, the transformation of the radioactive parent’s atomic nucleus ZXA into the daughter atom (Z+1YA) may be represented as below:
Fifth of the Laws of Radioactivity:
The number of atoms disintegrating per second of a radioactive sample at any time is directly proportional to the number of atoms present at that time.
The rate of disintegration of the sample cannot be altered by changing external factors such as pressure, temperature, etc.
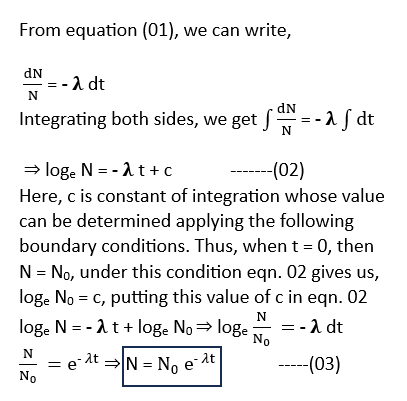
Let us suppose that a radioactive sample contains N0 atoms initially at (t =0). As time goes on, the atoms of the given sample decrease due to disintegration. Assume that the number of atoms reduced to N after time t and the number of atoms further decreased to N – dN after time t + dt. It is evident that in the time interval dt, the number of atoms decreases by dN
Therefore, the rate of disintegration of the atoms in the sample at time t* = dN/dt
Now, according to the radioactive decay in law, as mentioned above, the rate of disintegration at any time t is directly proportional to the number of atomic nuclei present in the sample at that time. *Laws of radioactivity*
(dN/dt) ∝ N ⇒ (dN/dt) = -𝛌N —(01)
Here, 𝛌 is constant of proportionality and is referred as the decay constant or disintegration constant, or e transformation constant of the given radioactive sample. The negative sign indicates that the number of atomic nuclei decreases as time elapses.*Laws of radioactivity*
The equation. 03 gives the number of radioactive atoms left alive after a time t. It means that the number of atomic nuclei of a radioactive sample decreases exponentially with the passage of time. On plotting a graph between N (the number of atomic nuclei left behind at time t) and the time t, the graph will be obtained as shown in [Figure 8.35]. The following conclusions can be drawn from this graph: *Laws of radioactivity*
1. Since, the decay of a radioactive sample obeys exponential law, the number of atomic nuclei falls rapidly in the beginning, and the rate of decay becomes slower and slower as the number of atomic nuclei decreases with the passage of time.*Laws of radioactivity*
2. The graph meets the time axis at Infinity. Therefore, all the radioactive samples, irrespective of their nature, will always take infinite time to disintegrate completely. In this respect, all the radioactive samples seem to be identical, however, this is not true. Some radioactive substances are found to be more active than others.*Laws of radioactivity*
3. The slope of the curve for this graph, indicates that the rate of disintegration of a radioactive sample is determined by the value of its decay constant(𝛌). A radioactive sample having a larger value of the decay constant (𝛌) will decay rapidly in that case the graph between N and t falls off rapidly with the time. *Laws of radioactivity*
Radioactive decay constant:
The decay constant, or disintegration constant, of a radioactive sample is a characteristic of the radioactive substance. The radioactive substances with a large value of the decay constant decay rapidly in comparison to those with a small value of the decay constant, according to the radioactive decay law.*Laws of radioactivity*
A fundamental quantity used in nuclear physics and radiochemistry to define the rate at which a radioactive substance undergoes decay is the radioactive decay constant (λ). It is a measure of the probability of an individual radioactive nucleus decaying per unit of time.*Laws of radioactivity*
Radioactive decay is a random process, and the decay constant quantifies the likelihood of a particular radioactive nucleus decaying within a specific time interval. Mathematically, the decay constant is defined as the reciprocal of the average lifetime (τ) of the radioactive nuclei. Thus, according to the decay law,*Laws of radioactivity*
(dN/dt) = -𝛌N or 𝛌 = [-dN/dt]/N
Therefore, the radioactive decay constant (𝛌)of a radioactive substance may also be defined as the ratio of its instantaneous rate of disintegration to the number of atoms present at that time. As is known,
N = N0 e–𝛌t , if t = 1/𝛌, then N = N0 e–𝛌./𝛌 = N0 /e = 0.368 N0
Hence, the radioactive decay constant of a radioactive substance may also be defined as the reciprocal of the time after which the number of atoms of the radioactive substance decreases to 0.368 or 36.8% of their number present initially at time t = 0. It is measured in Sec-1. *Laws of radioactivity*
half life time:
The half life of a radioactive substance is defined as time during which half of the atomic nuclei of the atoms of the radioactive substance will undergo disintegration. It is denoted by T.
Let us consider that a radioactive sample contains N0 atomic nuclei at t =0 (initially). Then, the number of atomic nuclei left behind after a time t is given by
N = N0 e–𝛌t ——(04)
As per the definition of half life of a radioactive sample, when t = T (half life), N = N0/2. Thus, the equation (04) will take the form
N0/2 = N0 e–𝛌T ⇒ e–𝛌T = 1/2 or e𝛌T= 2
𝛌T = loge 2 = 2.303 log10 2 = 2.303 x 0.3010 = 0.693
𝛌 = 0.693/T ——(05)
Therefore, from equation 05. it is evident that half life of a radioactive substance is inversely proportional to its decay constant and is a characteristic property of its nucleus. It can not be changed. by any knob methods like change in temperature and pressure of the radioactive specimen. *Laws of radioactivity*
The fraction of the atomic nuclei of a radioactive sample left behind after a time t in terms of its half life can be expressed in another form of relation. The number of atomic nuclei of a sample reduced to N0/2, implying that N/N0 =1/2 after one half life. After another time equal to T, the value of N/N0 will be (1/2)2 . In this way, after a time equal to n half lives, we can write,
N/N0 = (1/2)n = (1/2)t/T ——(06)
Mean life or average life:
The average time for which the atomic nuclei of a radioactive substance exists is called its average or mean life and is denoted by Ta. *Laws of radioactivity*
The mathematical expression of average or mean life of a radioactive sample by finding the total life of all the atomic nuclei of the substance in the sample and then dividing it by the total number of atomic nuclei present in that sample.
Let us suppose that the number of atomic nuclei present in a radioactive sample initially at time t = 0 is N0 and at time t, the number of atomic nuclei reduces to N. And further at time t + dt, the number of atomic nuclei are reduced to N – dN. It is evident that each of the dN atomic nuclei decaying during the interval of time t +dt has lived for a time t. This gives that the total life of dN atomic nuclei = t dN.

Therefore, average or mean life of a radioactive sample is equal to the reciprocal of its disintegration constant (λ).
The mean life or the average life and half life of a radioactive sample are related as T = 0.693 x Ta
Activity of radioactive substance:
The rate of radioactive decay of a radioactive substance is known as its activity. In order to become more stable, radioactive decay is the spontaneous process by which unstable atomic nuclei release energy in the form of radiation. The unstable nucleus changes into a new nucleus during this process, frequently one from a different element.
Usually, radioactive material’s activity is expressed in becquerels (Bq) or curies (Ci) units. One becquerel is equal to one radioactive decay event per second, while one curie is approximately 3.7 x 1010 becquerels. *Laws of radioactivity*
If N is the number of atomic nuclei present in a radioactive sample at any time t, then its activity at time t is defined as
R = – dN/dt —–(07)
The negative sign indicates that, the activity of the radioactive sample decreases as the time passes. Comparing the eqn. (07) with the expression of radioactive decay law, dN/dt = – 𝛌 N, we get *Laws of radioactivity*
R = 𝛌 N ——-(08)
Now differentiating, N = N0 e–𝛌t w.r.t time
dN/dt = – 𝛌 N0 e–𝛌t => R = 𝛌 N0 e–𝛌t
R = R0 e-𝛌t ——(09)
where, R0 = 𝛌 N0 represents the activity of the radioactive sample initially at t = 0.
Units:
(1) The practical unit of activity of a radioactive source is curie (ci). The activity of a radioactive sample will be one curie when it undergoes 3.7 x 1010 disintegrations per second. Thus, *Laws of radioactivity*
1 curie (ci) = 3.7 x 1010 disintegrations. s-1
Other multiple units are: 1 millicurie (mci) = 3.7 x 107 disintegrations. s-1
1 microcurie (µci) = 3.7 x 104 disintegrations. s-1
(2) The other unit of activity is called Rutherford (rd). The activity of a radioactive sample will be one Rutherford when it undergoes 106 disintegrations per second. Thus, *Laws of radioactivity*
1 rutherford (rd) = 106 disintegrations. s-1
Other multiple units are: 1 milli rutherford (rd) = 103 disintegrations. s-1
1 micro rutherford (µrd) = 1disintegrations. s-1
The relation between these two types of units of activity is: 1 curie (ci) = 3.7 x 104 rutherford (*Laws of radioactivity*)
Problems:
Q. 01
After a series of alpha and beta decays, Plutonium- 239 ( 94P239) becomes lead-207 (82Pb207). How many alpha and beta particles are emitted in the complete decay scheme? State the reason for your answer.
Answer:
Each time an alpha-particle is formed, the mass number of the radioactive parent’s nucleus, ZXA, is decreased by 4 and the charge number is also decreased by 2, while in beta-decay, mass number remains unchanged and the charge number increases by one unit.
The mass number of the parent nucleus, 94P239, decreased by 32 to transform into lead, 82Pb207. Thus, the number of alpha-particles emitted will be 32/4 = 8. Due to the emission of 8α-particles, the atomic number should decrease by 8 x 2 =16. therefore, atomic number should have become 94 – 16 =78. Because, the atomic number of daughter nucleus is 82. It is evident that plutonium must have emitted 4β-particles in order to conserve charge number as per nuclear reaction.
94P239—-> 82Pb207 + 8 2He4 + 4 -1e0
Q. 02
The half life period of a radioactive substance is 30 days. What is the time taken for 3/4th of its original mass to disintegrate?
Answer:
